Jackson Pollock couldn't possibly have been thinking of fractals when he started flinging and dripping paint from a stick onto canvas. After all, mathematicians didn't develop the idea of a fractal until a couple of decades later. But if one physicist is right, Pollock ended up painting fractals anyway. And that mathematical quality may explain why Pollock's seemingly chaotic streams of paint come together into an ordered, beautiful whole, and why the technique brought Pollock acclaim as a master of American abstract painting.
A fractal is a geometric structure in which the shapes at a large scale reflect the shapes at a small scale, forming an interlocking set of patterns that nest inside each other like Russian dolls. Approximations of fractal structures have been noticed throughout nature. For example, the overall crystal structure of a snowflake looks remarkably like the structure in a single arm. And the ridges of a mountain range jut into the sky, forming patterns similar to the crags thrusting out from a single peak.

|
| ©Pollock, Jackson, Number 1, 1950 (Lavender Mist), Ailsa Mellon Bruce Fund, image © Board of Trustees, National Gallery of Art, Washington
|
| PAINTING UNDER A MICROSCOPE. Fractals are objects that look the same under magnification as they do as a whole. One researcher says that Jackson Pollock paintings have that property.
|
In the same way, the web of large streaks of paint across a whole Pollock painting resembles the finer network covering a small section, Richard Taylor of the University of Oregon in Corvallis reported 8 years ago. He recently used these observations to investigate whether newly discovered paintings are really by Pollock, and hence worth millions of dollars, or whether they're destined for a garage sale. He proposes that the fractal nature of the paintings illuminates what made Pollock a genius rather than a mere slinger of paint.
Sexy results indeed-to some researchers, too sexy. Two scientists at Case Western Reserve University in Cleveland say that Taylor is stretching the mathematics too far to get his results. No fractals are lurking within the Pollock paintings, they say.
When Katherine Jones-Smith made some doodles on a page - "pretty ugly" ones, she says - she found that they shared the qualities of a Pollock, according to an analysis that follows Taylor's approach. "Either Taylor is wrong, or Kate's drawings are worth $40 million," says Jones-Smith's collaborator Harsh Mathur. "We'd be happy either way."
The attack by Jones-Smith and Mathur has sparked debate within the field and prompted a defense by the father of fractals, Benoît Mandelbrot, a professor emeritus at Yale University. "I have extraordinary experience of these structures," Mandelbrot says. Drawing on that experience, "I do believe that Pollocks are fractal," he concludes.
Geometrical fractalsAlthough it wasn't until 1975 that Mandelbrot developed the notion of a fractal, mathematicians were unknowingly stumbling upon fractals in the early 20th century. Helge von Koch, a Swedish mathematician, developed a curve that had some remarkable properties for calculus and was later recognized as a fractal. If you zoom in on any section of the curve, it looks precisely like the bigger section that contains it.
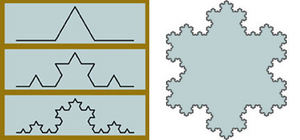
|
| ©I. Peterson
|
| A GEOMETRICAL SNOWFLAKE. The Koch curve was one of the first fractals discovered. To create one, start with a line segment, and then replace the middle third with a triangle. Then repeat, replacing the middle third of the resulting line segments with a tinier triangle (left). Keep going forever. Three Koch curves put together give a Koch snowflake (right).
|
Plus, as so often seems to be the case with fractals, the Koch curve is visually attractive, in a way that brings to mind objects from nature. When three sections of the Koch curve are put together into a rough circle, the pattern looks much like a snowflake.
Mandelbrot came upon fractals in 1961 when he was studying fluctuations in the cotton market. He noticed a surprising regularity: A plot of the seemingly random price variations over the course of a month looked just like a plot of the variations over a decade.
Soon, he was seeing such a pattern in remarkably many seemingly unrelated situations. The graph of the rise and fall of the Nile over a week resembled the graph over a century. The bumps and dips of the coast of Britain roughly resembled the irregular edge of a single cove. He dubbed objects with this pattern of self-similarity at different scales "fractal," from the Latin word for "broken" or "irregular."
Years later, the combination of visual beauty and geometrical precision of many fractals attracted Taylor, who had long been torn between physics and art. Alongside his career in physics, he created abstract art, even leaving physics for a time to study painting at the Manchester School of Art in England.
During that period, Taylor studied Pollock's paintings. The chaotic streams of paint seem far from the orderly precision of a Koch curve, but whenever Taylor looked at a small section of a Pollock painting, it looked similar to the overall structure of the whole.
The paintings didn't show the perfect self-similarity of a geometrical fractal like the Koch curve, but natural objects never do. Nature tends to improvise, provide variations on a theme, rather than repeat patterns exactly. A mountain range doesn't have precisely the same shape as the crags on a single mountain, but the two are similar. Taylor saw the same kinds of similarities in the Pollock paintings.
Taylor thought that the observation might help explain particular qualities. "Pollock's paintings are frequently described as appearing 'organic' and 'natural,'" Taylor says. "Pollock himself said that 'My concerns are with the rhythms of nature' and that 'I am nature.'" Was it the fractal feature of Pollock's paintings that created that organic quality?
Taylor pursued his idea by computing a statistic called the "fractal dimension," which mathematicians had developed to understand some of fractals' odd qualities. Ordinary lines with no breadth are said to have one dimension, and flat planes have two. But the Koch curve was puzzling. Since it is built from lines, it seemed as if it should be one-dimensional. But with more and more iterations of the curve, the line appears fuzzy, as if it had breadth. It couldn't be two-dimensional, though, because it doesn't fully fill any area.
So, mathematicians proposed a new notion of dimensionality - one in which the Koch curve is dimension 1.26. A less complex fractal would have a dimension closer to 1, and a more complex fractal would have a dimension closer to 2.
Taylor took a digital image of a Pollock painting into his lab, broke the image into its separate colors, and computed the fractal dimension of the lines in each color. Each time, he got a number between 1 and 2, confirming his notion that Pollock's paintings are fractal. "Rather than mimicking nature," Taylor says, Pollock "adopted its language - fractals - to build his own patterns."
In 1999, Taylor reported that the fractal dimension of Pollock's paintings increased during his life. His early drip paintings have a loose web of lines, mostly at the same scale. Because these paintings show no fractal qualities, their dimension is near 1. But Pollock's later paintings have a dense network of overlapping lines, ranging from large, bold strokes to delicate threads, Taylor calculated a fractal dimension of 1.72 for these works.
Taylor speculates that Pollock developed his artwork this way intentionally, "but on an intuitive, rather than an intellectual, level."
The challengeIn 2004, a graduate student in astrophysics at Case Western, Jones-Smith was to give a talk to her fellow students. "I was sort of bored with particle astrophysics," Jones-Smith says, so she looked around for something different. She came across an account of Taylor's work, and "it sounded really cool," she recalls.
"The obvious check to me was to make sure that not any old scribble would appear to be fractal," she says. "So, I made some scribbles." Much to her surprise, when she computed the fractal dimension of her scribbles, they turned out to be greater than 1.
Taylor's claim that the fractals in Pollock's paintings explained their aesthetic appeal doesn't hold up under mathematical scrutiny, Jones-Smith concluded. Mathur told her to publish her critique. But, since Taylor's research was 5 years old by that time, Jones-Smith decided that no one would care about her analysis. She dropped it and continued with her astrophysics work.
Last February, however, she read a story about Taylor in the newspaper. Back in 2002, Alex Matter found among his deceased parents' belongings a cache of 32 works that were painted in Pollock's drip style. Matter's parents had been friends with Pollock. Matter also found a note in his father's handwriting that said that the paintings were a "gift + purchase." If the paintings were genuine Pollocks, the find would be like a winning lottery ticket. One of Pollock's paintings, "No. 5, 1948," sold recently for $140 million.
The Pollock-Krasner Foundation, which handles Pollock's estate, turned to experts to determine the authenticity of the paintings. Taylor was asked to use his fractal analysis to give objective, scientific input.
When Taylor analyzed the new paintings, he found that none showed the fractal characteristics of many of Pollock's other paintings. Taylor will report his findings in an upcoming Pattern Recognition Letters. He cautions that his findings aren't definitive but should be considered with reports from other experts.
When Jones-Smith read the newspaper story about the analysis, she was dumbfounded. "What good is it to use Taylor's criteria to authenticate Pollocks if they can be imitated with such ease?" she asks. So, she and Mathur decided to go public with her critique. It was published in the Nov. 30, 2006 Nature.
Jones-Smith and Mathur argue that there's a fundamental problem with Taylor's work. Geometrical fractals show similar patterns at any degree of magnification: No matter how far you zoom in on the Koch curve, it looks the same. No natural object can match that. After all, the molecular structure of a pebble has no particular resemblance to the structure of an entire mountain range. But natural objects that are considered fractal do show similar patterns across several orders of magnitude. There's been substantial debate about how many orders of magnitude are necessary for something to properly be considered fractal.
Pollock's paintings show fractal qualities over too small a range of orders of magnitude, Jones-Smith and Mathur say. "Everything looks fractal if you look over such a small range," Mathur says.
Taylor retorts that in his analysis, Jones-Smith's doodles don't have the same fractal characteristics that the Pollock paintings do. Furthermore, he says, the paintings show fractal patterns over a range of orders of magnitude consistent with other fractal research. Using the standards they advocate, "Jones-Smith and Mathur would also dismiss half the peer-reviewed published investigations of physical fractals," Taylor says.
"That is true," Jones-Smith says. "We would not be uncomfortable dismissing over half of the published accounts. We feel as though the term fractal is used overzealously."
The community weighs inJones-Smith has jumped into a decade-old argument. David Avnir of the Hebrew University of Jerusalem in 1998 criticized much fractal research on the grounds that most natural objects under study as fractals show fractal behavior over too small a range. He argues that Taylor shouldn't use the word fractal to describe Pollock paintings, so that word can be reserved for geometrical fractals and the few physical objects that show fractal behavior over a large range of scales.

|
| ©Jones-Smith
|
| FRACTAL FOOLERY. Katherine Jones-Smith made this doodle, "Pebbles," which she says shows the same fractal patterns that Taylor has found in Pollock paintings. Therefore, she argues, the paintings aren't fractal.
|
But Lazaros Gallos, a fractals researcher at the City College of New York, says, "What [Jones-Smith and Mathur] have done is just a simple trick," Gallos says. "This is bad science about fractals."
Gallos explains, "There's not a well-established definition of fractals. When we try to give a definition, we mainly say that when you zoom in or out, it looks very similar to the whole." There's a strong indication that Pollock's paintings are fractal, he says, because they look fractal, and Jones-Smith's aren't because they don't.
Once the structure of an object has been determined to be fractal, the fractal dimension can be used to analyze that structure, Gallos says. But computing the fractal dimension of objects that aren't fractal is meaningless. "In practice," he says, "no matter what shape you take, you'll definitely get a non - integer fractal dimension."
Jones-Smith maintains that assessing whether or not a painting is fractal on the basis of appearance is subjective and unscientific. "I personally don't see small structure mirrored in large structure in Pollock's paintings," she says. "They look like a complete mess, as far as I'm concerned."
For authentication, it doesn't matter whether it's legitimate to call Pollock paintings fractal, says Michael Barnsley of Australian National University in Canberra. Taylor has a reproducible technique that produces numbers from a painting, and he can correlate those numbers with different artists. "That doesn't allow you to authenticate or not authenticate a painting, but you could certainly add it into the collection of information that you have to say that it's more likely," he says.
Jones-Smith argues that Taylor has studied only 17 paintings out of Pollock's 180 works, and that they are the most famous ones, not a representative sample. So, it's not clear, she says, whether the patterns that Taylor has seen hold up across Pollock's work.
Taylor says that he hopes to analyze the remaining paintings and that he's also planning to apply different fractal techniques.
Jones-Smith and Mathur also intend to analyze some of Pollock's other paintings, looking for ones that don't fit with Taylor's analysis. Furthermore, Jones-Smith plans to make some drip paintings to see whether her inartistic renderings will have the characteristics that Taylor says are typical of Pollock's works.
So far, the Pollock-Krasner Foundation has made no official decision about the authenticity of the works. But on Jan. 29, a group at Harvard University released a study analyzing the pigments used in the paintings. Its results agreed with Taylor's suspicions about the paintings. The Harvard group found that the pigments weren't commercially available until years after Pollock's death in 1956.
Hany Farid, a computer scientist at Dartmouth University in Hanover, N.H., who has worked on art authentication, says that no matter how the quarrel over the fractal nature of Pollock's work turns out, Taylor's research points at something deeply true.
"The difference between math and art is not as great as people think," Farid says. "There is an art to mathematics and a mathematics to art."
Reader Comments
to our Newsletter